- Conic Section Art - Mrs. Miller's Precalculus Projects This teacher had students create pictures using conic sections. They must include the equations for each conic section used. This is a great idea to.
- A Conic Section Compass can continuosly trace conic sections exploiting the very definition of it. In this particular build the pencil lays on the surface of a 30 degrees cone (but it could be any other angle) and can freely slide in the pencil holder: in this way it will always be in contact with the intersection surface (the paper sheet).
- I have assigned conic picture projects wayyyy before Desmos. My students did them by hand in the old days, and even on TI-83's (not easy) after that. This is the first time I ever did the project using Desmos, and it was a huge success. My students exceeded my expectations. Here is the project, some of which I got Mr.
Precalculus Projects. Circular Trig Art. Grading Rubric for Circular Trig Art. Conic Section Art. Conic Section Art Directions. Precalculus Projects .
One of the hallmarks of the MTBoS is constant refinement and reflection – taking something of your own or someone else’s and making it better.
The conics unit has come and gone in my Algebra II classes, and like last year I want to do a performance assessment. Back in the day this assessment was Amy Gruen’s piecewise functions picture. With the advent of Desmos it’s now a digital version of the same project. (I wrote about last year’s here). Then in early summer I saw the tweet that let me know how much better my project could be for my students.
I am so stinking proud of my Alg students' @desmos projects! #mtbos#mathartpic.twitter.com/03gNDfEN7o

— Annie Perkins (@Anniekperkins) June 12, 2017
Dropping the image into Desmos first, then creating the equations to match the image? Brilliant! That led to a pretty productive online conversation, and to me making some slight changes to my plan for this year. My big takeaways from last year were:
- my students selected some very cool but also very challenging pictures to duplicate
- they needed massive amounts of support writing equations to match lines and curves
- probably not everybody did their own work
Providing massive amounts of support is what Desmos does best. That scaffolding probably means less frustration, and less cheating. At least that’s what I’m telling myself.
Started before break with a functions review (Alg II (3) Functions one-pager), not only of conics but of all the functions we’ve learned this year. The day back from spring break we learned how to match equations with lines or shapes in a picture with this Desmos activity.
Then I introduced the project, and offered a carrot (it’s a quiz grade, you guys!). And away they went, seeking pictures.
This slideshow requires JavaScript.
They found standard-issue high-school-kid stuff: lots of cartoon characters, superhero or sports team logos, palm trees and flowers. I had them make a (rough) sketch of the image on grid paper, then try to identify equations of four functions that would be included in the final product. I wanted them to get used to the idea of seeing small sections of the larger whole, and finding ways to describe that section in math symbols. We also walked through the process of setting up an account in Desmos, opening a new graph and bringing in the image, and saving the graph so they could access it again.
By Day Two, we were ready to start getting serious about making some math art.
They were pretty excited about this project when they were googling around for images, finding their favorite characters or sports teams. They were less excited about this project when it came time to start writing equations.
Dearest Martha:
I fear a low-grade panic is setting in amongst the troops as they face the challenge of the Desmos Art Project. They are despairing of ever being able to write equations to match shapes. We are headed for crushing defeat unless I can rally them. #teacherlifepic.twitter.com/W07Xuac4K3
Conic Art Project With Equations Pdf
— Stephen Dull (@thedullguy) April 5, 2018
A couple wanted to straight-up quit. I’m gonna use all my powers of persuasion to try to convince them otherwise. That, plus walking through the process, step by-step, of writing a general equation, then adding sliders and tweaking values until the curve matched up. I’m not sure it helped.
I did notice that very few of my students actually completed the reference sheet. And (in a related story) almost none had any recall of any function equations except y = mx + b. That is definitely part of the issue – a huge disconnect between a shape on a screen and the math symbols that represent it. And truth be told, that’s part of what I wanted this assignment to do – to cement that relationship.
Best-laid plans, right? I’ve got some work to do.
The morning of Day Three, the putative due date, one of my struggling students came in for extra help on the project. She left with a smile on her face, having made serious progress. Plus she agreed to act as a “resident expert” in class, helping out her tablemates when they got stuck. We made some halting progress as a class, but no one is close to done. Several of my students did say that they understood how to write an equation for a line or curve, and restrict the domain, just that it was going to take a long time and a lot of tedious work. So, similar to last year, with about 10 minutes left in class I offered a reprieve, shifting the due date to Monday. Then I’ll accept whatever they have and go from there. I set up the grading rubric in such a way that the points are weighted toward planning and less on the finished product, so the kids who laid down a foundation can still get a reasonable grade even if their final product is…. incomplete.
But I also want to be able to show them what their project could look like, with a little bit of persistence:
This slideshow requires JavaScript.
Just a little something I threw together over the weekend. 44 equations later…
The breakthrough for many came when they started to use vertex or intercept form for their parabolas. The ones who completed the functions reference sheet caught that first. I showed everyone on Monday, which of course was too late for many folks. Next year I’ll highlight that option earlier.
So, they begrudgingly turned in their paper/pencil planning work, along with a link to their Desmos creation, on Monday. Just like last year, some bit off way more than they could chew. Some got frustrated and quit. Some gave me a half-finished product. But the ones who stuck with it were able to turn in some pretty cool stuff:

This slideshow requires JavaScript.
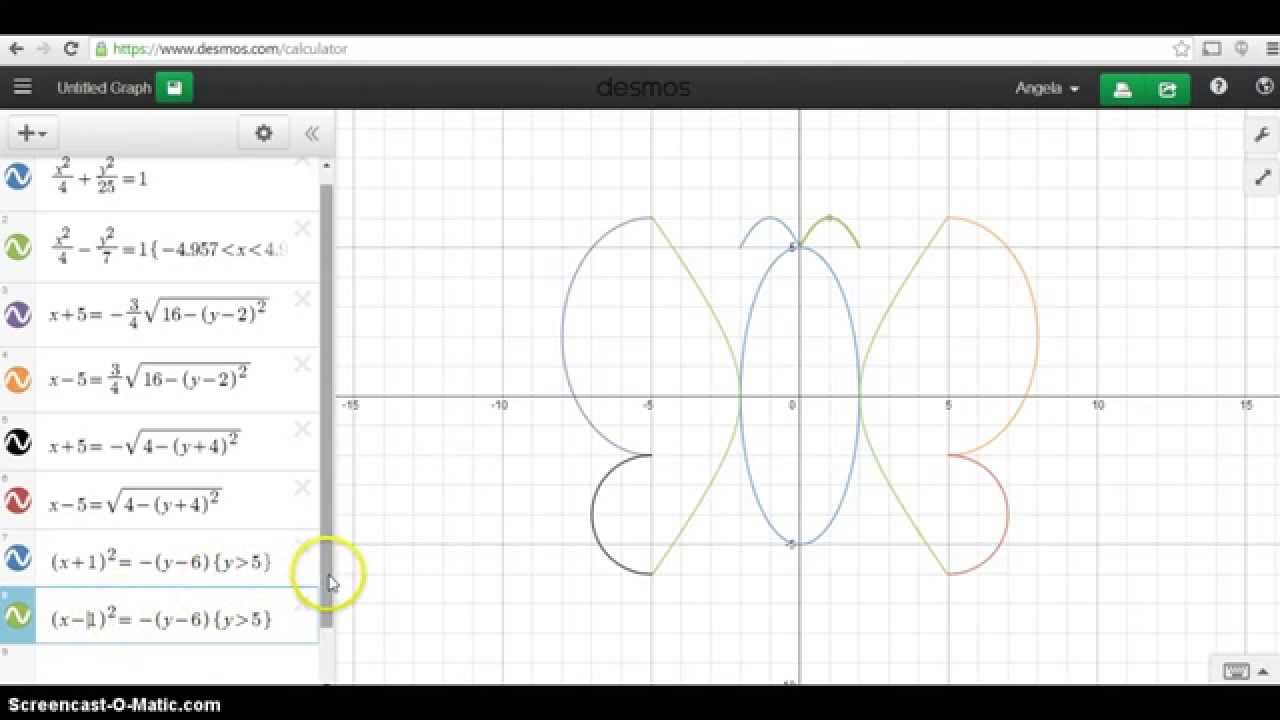
Oh, yeah, and this from a student as she turned in the assignment thru Canvas:
My big takeaways:
Hyperbola Conic Equation
- I need to steer them towards reasonable images to duplicate. Avoid frustration and shutdown right from the jump.
- I need to encourage my students to use the vertex form of quadratics. Anything that makes the movement of the curve more intuitive is good. I think eventually that will help cement translation of functions.
- I need to enforce the preparation steps that I built in: the reference sheet, the paper sketch, and the four function equations by hand. I need to help them draw the connection between curves on a screen and the associated math symbols.
The assignment is is a keeper. But I bet you it won’t look exactly the same three years from now as it did this week. In fact, I’m counting on it.